The image is from the Giza Plateau Computer Model.
The largest of the Giza pyramids is usually called the Great Pyramid.
I prefer to call it the Great Golden Pyramid, because its geometry
is that of the Golden Mean
PHI = (1 + sqrt(5))/2 = 1.618... .
The Great Golden Pyramid has height sqrt(PHI) = 1.272...
and base 1+1 = 2.
Each triangular face intersects the ground at an angle
of arcsin(sqrt(PHI)/PHI), a little less than 52 degrees.
Since 4/sqrt(PHI) = PI, approximately, so that the circumference
of a circle whose radius is the height of the pyramid is
2 x PI x radius = 2 x PI x sqrt(PHI) =
= 2 x (4/sqrt(PHI)) x sqrt(PHI) = 2 x 4 = 8 ,
and since the perimeter of the base of the pyramid is
2 + 2 + 2 + 2 = 8 ,
the Great Golden Pyramid approximately squares the circle.
Exterior measurements of the Great Golden Pyramid are
now very uncertain, because the casing of limestone blocks
has been removed over the years.
Some smaller blocks are in European museums,
but most of them are in Cairo,
where they are now part of dams, bridges,
and mosques (such as the Sultan Hasan Mosque).
Each side of the base of the Great Golden Pyramid
is about 440 Royal Cubits (one RC = about 0.524 meters),
so that each semibase is 220 RC,
the total perimeter of its base is about 8 x 220 = 1760 RC.
Its height, if it had a capstone, would be about 280 RC.
Therefore, the ratio of perimeter to height
would be approximately PI: 1760/280 = 3.14... .
Each face is an isosceles triangle of height PHI times the semibase.
Such an isosceles triangle has central angle
2 arccos(PHI/sqrt(1+PHI^2)) = 63.43... degrees.
If three such triangles are used to make
a pyramid (instead of the four at Giza),
and if they are mirrors to make a
tetrahedral mirrorhouse,
the result is an icosahedral kaleidoscope.
In describing the Great Golden Pyramid,
I do not try to be exact about lengths, angles,
and such, but only approximate. One reason for
discussing it approximately is that the pyramid
itself has been disturbed by some earthquakes
over the last few thousand years:
This gif image amplifies the magnitude of the disturbance
by a factor of 10 to show it more clearly.
Another reason is that my astronomical software
does not go back in time to 9000 BC, so I have
just estimated with a paper precession chart,
a 6-inch star globe, and protractor, ruler, and compass,
to see roughly what is where.
Still another reason for using rough estimates is
my general attitude that you can get a better
overall view of something by initially making
rough overall estimates than by trying to
achieve a lot of precision at first.
Rough first approximations can often show
you what is important and what is not,
and then later you can refine the important things.
The Great Golden Pyramid contains two interior chambers
and a number of interior shafts. The interior shafts
all generally lie in a north-south vertical plane
section of the pyramid, displaced a little east of
the center of the pyramid:
It (like many other Egyptian pyramids) has chambers underneath,
but the Great Golden Pyramid is the only Egyptian pyramid
with interior chambers.
The higher interior chamber, usually called the King's chamber,
is called here the Upper Chamber.
The lower interior chamber, usually called the Queen's chamber,
is called here the Mid Chamber.
The Entrance shaft starts at an entrance on the north face,
a little above ground level,
and descends at an angle of about 26 degrees.
Since the latitude of Giza is about 30 degrees North,
if you go into the entrance shaft and look back,
you will see a part of the sky centered about 4 degrees
from the North pole in the sky.
If you could see through the ground and the Earth as
you looked forward going down the Entrance shaft,
you would see an area near the South pole in the sky.
WHAT STARS WERE AT THE NORTH POLE WHEN THE GREAT GOLDEN PYRAMID WAS BUILT? Since I think that the Great Golden Pyramid represents the Supernova Vela X of about 9000 BC, I think that it was built at that time. Due to the precession of the equinoxes (a 26,000 year cycle stabilized by the moon and sun)
the north pole was in the constellation Hercules,
near the head of Draco the Dragon.
The Egyptians identified Draco with a crocodile,
the Typhon or card 15 of the Tarot Major Arcana.
The Entrance shaft continues down to the conventional chamber under (not inside) the pyramid. However, part way there an Ascending shaft branches off, and goes up to the two interior chambers. That shaft goes up at a slope of about 26 degrees. If you could see through the pyramid to the sky in its direction, you would see (at a certain time of day) the Belt of Orion. At another time of day, you would see the Southern Cross. The height of the entire pyramid (not including the capstone, which is and has been missing) is 203 courses, or layers, of varying thicknesses, totalling about 264.5 RC. If a capstone went to the theoretical apex of the pyramid, the total height would be about 282 RC, or, roughly rounding, 280 RC. At a little bit above the 25th course, the Ascending shaft branches into 3 passages: the Well Passage, descending vertically; the horizontal branch to the Mid Chamber; and the continuation into the Grand Gallery.
The Well Passage is a vertical descending branch,
leading through an irregular shaft down
to the chambers underneath the pyramid.
The horizontal branch leads to the Mid Chamber,
which is located on the East-West plane of the pyramid
just East of the North-South plane.
The Mid Chamber is made of limestone,
10 RC in the East-West direction,
11 RC in the North-South direction,
and the height of the North and South side walls
is (20/sqrt(5)) RC = about 8.94 RC.
The height of the top of the gable roof is about 11.8 RC.
A 5-step corbeled Niche, from 1 RC to 2 RC deep,
from 1 RC to 3 RC wide, and (20/sqrt(5)) RC = about 8.94 RC high,
has been carved in the East wall.
The top of the side walls is 50 RC above the base of the pyramid.
A crust of salt is on the walls of the Mid Chamber.
Two small shafts go North and South from the Mid Chamber
at an angle of about 39 degrees.
Their openings were sealed until about 1872.
The top of the openings are about 3.3 RC from the floor,
the same level as the top of the bottom section of the Niche
and the roof of the entrance into the Mid Chamber.
The Southern shaft has been explored by a robot used
by Rudolf Gantenbrink of Munich. He has found it to be
blocked by a stone with handles. The Egyptian government
may explore it further in May 1996.
Since the 39 degree angles of the Mid Chamber shafts
are nearly the right-angle-complements of the 52 degree
angles of the faces of the pyramids with the ground,
the Mid Chamber shafts symbolically place the Mid Chamber
at the apex of an inverted intersecting and interpenetrating
Great Golden Pyramid.
In this way, the Mid Chamber (which is smaller than a
capstone that would complete the geometry of the pyramid)
might be regarded as being the interior chamber of a "virtual" capstone,
and the Niche might be a "door" to a "virtual gallery shaft"
leading to the "outside" of the "virtual" capstone.
Returning to the Ascending shaft, and continuing ascent,
you enter the Grand Gallery, an upward extension continuing
to ascend at the 26 degree slope toward the Antechamber
preceding the Upper Chamber.
The Grand Gallery has 7-step corbeled side walls,
2 RC wide at the top and 4 RC wide at the floor.
The floor has a central groove 2 RC wide,
flanked by 1 RC floor-ramps on each side.
The Grand Gallery is about 18 RC high vertically,
or about 16 RC high perpendicular to its floor.
It is about (200/sqrt(5)) = about 89 RC long.
There are 55 ramp-holes on the sides of the floor-ramps.
Some parts of the Grand Gallery walls contain salt deposits,
but not as much as in the Mid Chamber.
At about the level of the 50th course of the pyramid,
the Grand Gallery reaches the level of the Upper Chamber
and leads through a low passage way about 2 RC x 2 RC x 2.5 RC
to the Antechamber of the Upper Chamber.
The Antechamber is about 5 RC long, 2 RC wide and
a little over 5 RC high.
Its side walls have 4 pairs of vertical grooves,
each about 4 RC high.
3 go all the way to the floor.
The first pair of grooves stop 2 RC above the floor,
and contain a two-section granite leaf about 2 RC high,
each section being about 1 RC high.
You could slide the top section of the granite leaf
up and out of the first pair of grooves,
then move it over and drop it into
one of the other 3 pairs of grooves.
You could then do the same thing with the bottom section.
In this way,
you could make a 2 RC high wall in any one
of the other 3 pairs of grooves,
or you could make two 1 RC high walls in any two of them.
Such a 2 RC high wall in the last pair of grooves
would block the entrance to the Upper Chamber,
which is through a low passage way about 2 RC x 2 RC x 5 RC.
Other such configurations would not block the entrance,
but you could use them to act as "dams" to contain
water or other liquids in the Upper Chamber
and the Antechamber.
The Upper Chamber itself is made of granite,
about 10 RC wide (North-South),
about 20 RC long (East-West),
and about 5 x sqrt(5) RC = about 11.18 RC high.
The diagonal of each North-South wall is about 15 RC.
The diagonal of the whole chamber is about 25 RC.
The ratio of the North-South wall diagonal
to the East-West length to the whole chamber diagonal
is about 15:20:25 = 3:4:5 .
A granite coffer is inside.
It is about 2 RC high, about 1.9 RC wide, and about 4.36 RC long.
It is too wide to be taken in or out through the Entrance shaft.
Two small shafts go North and South from the Upper Chamber.
The top of the Northern shaft is about 2 RC above the floor.
The shaft goes up at an angle of about 32 degrees to exit
the pyramid at the 101st course.
If you looked out the Northern shaft,
you would see the area of the North polar sky.
If you went outside the pyramid and looked down the shaft,
and could see through the pyramid and the Earth,
you would see the area of the South polar sky.
The top of the Southern shaft is also about 2 RC above the floor.
The shaft goes up at an angle of about 45 degrees to exit
the pyramid at the 102nd course.
If you went outside the pyramid and looked down the shaft,
and could see through the pyramid and the Earth,
then (at a certain time of day) you would see
the Center of the Milky Way galaxy in Saggitarius.
If you looked out the Southern shaft from within,
then (at the same certain time of day) you would see
the thinnest part of the Milky Way,
North of Betelgeuse, between Orion and Taurus.
Above the roof of the Upper Chamber are 5 Ceiling Chambers.
They are formed by 5 granite slabs
and a 6th top roof of gabled limestone.
They were opened by excavations by Vyse in the 1800s.
The lowest of the 5 Ceiling Chambers was found
to contain a black dust, possibly the remains of
insects entombed in construction.
The limestone gable of the highest chamber is the only place
(other than the Mid Chamber and some parts of
the Grand Gallery) that contains salt deposits.
The Ceiling Chambers contained some markings that
were, in my opinion, put there at the time of the
exploration by Vyse in the 1800s.
If the 6 slabs are considered to be lines of
an I Ching hexagram,
with granite slabs being unbroken lines
and a limestone gable being a broken line,
you get the hexagram Lake over Heaven,
which can be interpreted as -
Bad news comes, you should depart.
WHAT IF - in order to depart this Earth,
you create a Lake in the Upper Chamber
over the Heaven of the "virtual" capstone Mid Chamber -
so that:
the Upper Chamber is sealed off by
the granite leaf sections of the Antechamber,
with the Upper Chamber containing a liquid;
the Ceiling Chambers contains reacting
chemicals that drained down into the Upper Chamber;
the reactions produces gases, radiation, whatever,
which could only exit the Upper Chamber by way of
the Northern shaft (to the North polar region)
because the Southern shaft
(to the thin part of the Milky Way to the North of Orion,
or to the center of the Milky Way in Saggitarius)
was blocked at the time the Great Golden Pyramid was built
by a gold-plated non-meteoritic iron plate about 0.5 RC by 0.5 RC,
located a few meters from the outer surface of the pyramid;
after the reactions have run their course,
the granite leaf sections are moved,
allowing the spent reactants and liquid to
drain away down the central groove of the floor
of the Great Gallery to the Well Passage,
and thence to the chambers underneath the pyramid,
where they soak into the surrounding earth;
with some of the spent reactants and liquid
spilling over into the passageway to the Mid Chamber,
which might, as the liquid evaporates over time,
accumulate salt deposits.
In his 1973 book, Tompkins states: "Petrie found no evidence of hollowing along the lower-level casing stones, running along the base of the Pyramid, which have now been completely uncovered. A recent survey by two Italian scholars, Maragioglio and Rinaldi, indicates the casing stones ABOVE the base line may have been slightly sloped toward a central line." Since the casing base-line has no hollowing, each casing face cannot be divided into only 2 triangles, but can be divided into 3 triangles. My ideas about the geometry of the 3 triangles on each face of the Pyramid are a result of e-mail correspondence with Terry Nevin. Since each face of the Great Golden Pyramid is only an isosceles triangle, not equilateral, it is not possible for all 3 triangles on a given face to be equivalent. However, it is possible to make them equal in area, and that is what I do here. Here is a face-on side view of the Great Golden Pyramid showing 3 equal-area triangles per face:
Here is an edge-on side view of the Great Golden Pyramid
showing 3 equal-area triangles per face:
Here is a top view of the Great Golden Pyramid
showing 3 equal-area triangles per face:
The Great Golden Pyramid is shown as being truncated
because it may never have had a conventional capstone.
Some special object,
like the eye above the pyramid on the US dollar bill,
could have been located on the approximately 14.5 meter square top
at course level 203, about 9.26 meters below the theoretical apex.
Since the Great Golden Pyramid squares a circle,
and is so associated with a hemi-sphere
centered on the center of its base,
you can ask at what "latitude" on the hemi-sphere
is the intersection point of the 3 face triangles.
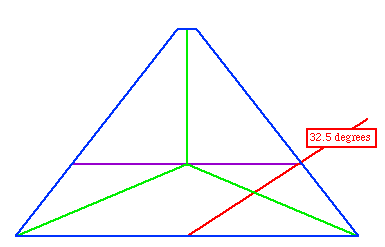It is about 32.5 degrees,
about 2.5 degrees north of the latitude of Giza,
and the same angle as the north shaft of the Upper Chamber.
Therefore, the intersection point of the 3 triangles
of the north face point in the same direction
as the north shaft of the Upper Chamber,
approximately to the celestial north pole.
This might support the ideas of Bauval and Hancock that
the Great Golden Pyramid's associated hemisphere might
be a representation of the Earth.
The top platform would be a square
of side somewhat less than 6 degrees,
so that if represented the point on Earth
with longitude of its location, Giza,
and with latitude of 32.5 degrees,
it would include Giza, at latitude 30 degrees,
and also a region of the Eastern Mediterranean area
roughly from Giza to Cyprus.
The geography of the Giza Hemisphere
is roughly sketched above.
The North Pole is beyond Leningrad from Giza.
Cahokia is beyond London from Giza.
Tokyo is beyond Xi'An from Giza.
Andaman refers to the Andaman Islands beyond India.
The Cape Verde Islands are beyond North Africa.
The Brazil Basin is in the North Atlantic Ocean off South America.
From South Africa, the East Africa Rift Valley
goes north through the Highlands near the Nile Valley towards Giza,
turning back southeast from the Red Sea down through
the Indian Ocean to the SE Indian Ridge
between Australia and Antarctica.
As Bauval and Hancock have noted,
the scale of the Giza Hemisphere is 1 to 43,200.
Since 43,200 = 600 x 72,
it may be related to
the 4-dimensional version of the 3-dimensional icosahedron,
the 600-cell whose construction involves the Golden Ratio,
and
the 72 root vectors of the E6 Lie algebra
used in the D4-D5-E6 physics model.
The outer casing of finished limestone of the Great Golden Pyramid
has long since been removed,
leaving its core-masonry as what now appears as the outer faces.
The core-masonry outer faces are NOT flat,
but are hollowed.
The dotted line in the figure above indicates
the concavity of the casing hollowing,
which would be hidden in the complete Pyramid,
but is now visible:
The core-masonry of the Second and Third Pyramids
are also exposed, but are clearly flat, not concave,
so the concavity was not a building technique:
it must have symbolized something.
WHAT could be symbolized by
the 3-triangle outer casing faces?
Mathematically, the pattern looks like
the Dynkin-Coxeter diagram of the D4 Lie algebra Spin(0,8)
and like
an octonion multiplication diagram.
WHAT could be symbolized by
the concave hollowed core-masonry base?
The plan of the Giza plateau,
including the 3 Pyramids and their 2 vanishing points,
was constructed based on the elliptic spherical geometry
connecting structures on a 2-dimensional Earth surface
with stars on a 2-dimensional celestial sphere.
The base of 4 concave faces could represent hyperbolic geometry
as the red region in the center of the hyperbolic Poincare disc.
In hyperbolic geometry, as in MInkowski spacetime,
time and space are represented differently.
That is not the case in elliptic spherical geometry,
in which space and time are indistinguishable.
This can be expressed by saying that
the elliptic spherical metric has signature ++...
while the hyperbolic metric has signature -+...
(where ... indicates all the spatial dimensions have + signature).
Consider the case of 2 dimensions, 1 time and 1 space.
Elliptical spherical geometry is the geometry of
circles on a sphere, and all the circles look alike.
Hyperbolic geometry is the geometry of
a disc whose boundary is a circle.
The disc is made up of two different kinds of things:
_______
The timelike things are arcs of circles
that are perpendicular to the boundary circle.
4 of these form the boundary of the red region
in the figure above (by Juha Haataja at CSC).
In higher dimensional spacetime,
these arcs would still be 1-dimensional arcs.
The spacelike things are circles inside the disc that
are tangent to the disc at its boundary circle.
They are called horocycles. In higher dimensional spacetime,
the horocycles would be spheres instead of circles.
If you look only at a spacelike horocycle in
the hyperbolic spacetime, you see that,
since it is a sphere, it has spherical geometry.
In N-dimensional hyperbolic geometry,
spacetime is divided into 1-dimensional time
and
spherical (N-1)-dimensional space.
If I represents an interval of real numbers
(such as zero to (but not including) pi)
and if S3 is the 3-sphere and S7 is the 7-sphere,
then I x S3 or I x S7
could be a hyperbolic geometric representation
of the spacetimes of the D4-D5-E6 physics model.
As Euclidean geometry is intermediate between
elliptic spherical geometry and hyperbolic geometry,
the Pyramids at Giza show all 3 types of geometry:
The plan of the 3 pyramids on the Giza plateau,
on the surface of the spherical Earth, has SPHERICAL GEOMETRY;
Each pyramid itself is a 4-sided pyramid, and has EUCLIDEAN GEOMETRY.
If there were a mirror image pyramid below the physical pyramid
that is above ground, they would not form an octahedron
because the faces are not equilateral triangles. They would
form a 4-sided bypyramid, whose symmetry group is the D4
finite group corresponding by the McKay correspondence to Spin(8).
The horizontal cross-sections of the core-masonry
of the Great Pyramid has HYPERBOLIC GEOMETRY.
If the Great Pyramid is regarded as a bunch of
horizontal hyperbolic planes stacked up vertically,
or H2 x I where H2 is the hyperbolic plane and I is an interval,
then its resulting geometry is an example of a
3-dimensional geometry that is homogeneous but not isotropic.
Any horizontal section of such a Great Pyramid would
have hyperbolic geometry, but
any vertical cross-section (such as the plane of its chambers and shafts)
would have Euclidean geometry.
Spherical, Euclidean, and Hyperbolic geometries are all
subgeometries of LIE SPHERE GEOMETRIES.
Lie sphere geometries are the geometries of hyperspheres
embedded in Spherical space, Euclidean space, or Hyperbolic Space.
As homogeneous spaces, ordinary spheres Sn are
Spin(n+1) / Spin(n)xSpin(1)
where Spin(1) is the 2-element group {-1, +1},
and
Lie spheres are Spin(n+2) / Spin(n)xSpin(2)
where Spin(2) = U(1).
Since it uses such structures as Spin(10) / Spin(8)xU(1)
the D4-D5-E6 physics model
can be said to be based on Lie sphere geometry.
More interesting math (and language) stuff is on an Egyptian Hieroglyphics page.
REFERENCES: Many of the facts and images are taken from the following references - (however, I am responsible for any erroneous speculations): Bauval, R., The Orion Mystery (Crown, 1994, 1995); Bauval, R. and Hancock, G., The Message of the Sphinx (Crown, 1996); Cecil, T., Lie Sphere Geometry (Springer-Verlag, 1992); Hancock, G., Fingerprints of the Gods (Crown, 1995); Helgason, S., Groups and Geometric Analysis (Academic 1984); Huang, K., and Huang, R., I Ching (Workman, 1987); Kappraff, J., Connections (McGraw-Hill, 1991); Kaufmann, W., Universe (Freeman, 1988, 1991, 1994); Lemesurier, P., The Great Pyramid Decoded (Element, 1977, 1993); Lemesurier, P., The Great Pyramid, Your Personal Guide (Element, 1987); Levy, S., Automatic Generation of Hyperbolic Tilings, in The Visual Mind, Emmer, ed. (Leonardo 1993); Montesinos, J., Classical Tessellations and Three-Manifolds (Springer-Verlag 1987); Tompkins, P., Secrets of the Great Pyramid (Allen Lane 1973); Weeks, J., The Shape of Space (Marcel Dekker 1985).